1. Main temporary changes
- Incorporating the merging of local authorities
- Changes to the international migration component
3. Temporary method changes
Incorporating the merging of local authorities
The three main sources of uncertainty associated with the mid-year population estimates (MYEs) are the census base, international migration, and internal migration (moves between local authorities (LAs)). Uncertainty in the other components of change (births, deaths, asylum seekers, armed forces, and prisoners) is assumed to be zero.
The methodology for producing internal migration uncertainty has remained the same for local authorities whose geographic boundaries have not changed in recent years. Further details on this process can be found in Methodology for measuring uncertainty in ONS local authority mid-year population estimates: 2012 to 2016. In 2019 and 2020, a number of local authorities merged into new ones. Table 1 outlines which local authorities were affected. Because of certain features intrinsic to the internal migration methodology, it is not possible to process them the same as other local authorities.
| Old local authority | New local authority | |||
|---|---|---|---|---|
| LA code | LA name | LA code | LA name | Year of boundary change |
| E06000028 | Bournemouth | E06000058 | Bournemouth, Christchurch and Poole | 2019 |
| E07000048 | Christchurch | |||
| E06000029 | Poole | |||
| E07000053 | Weymouth and Portland | E06000059 | Dorset | 2019 |
| E07000052 | West Dorset | |||
| E07000050 | North Dorset | |||
| E07000051 | Purbeck | |||
| E07000049 | East Dorset | |||
| E07000191 | West Somerset | E07000246 | Somerset West and Taunton | 2019 |
| E07000190 | Taunton Deane | |||
| E07000205 | Suffolk Coastal | E07000244 | East Suffolk | 2019 |
| E07000206 | Waveney | |||
| E07000201 | Forest Heath | E07000245 | West Suffolk | 2019 |
| E07000204 | St Edmundsbury | |||
| E07000004 | Aylesbury Vale | E06000060 | Buckinghamshire | 2020 |
| E07000005 | Chiltern | |||
| E07000006 | South Bucks | |||
| E07000007 | Wycombe | |||
Download this table Table 1: Boundary changes to local authorities 2019 to 2020
.xls .csvWe calculated the relative width and position of the uncertainty intervals for each LA in the newly merged LAs. We combined them into a weighted average to produce uncertainty intervals for the 2020 MYE in the following manner:
1.
2.
Where MYE2019 is the 2019 mid-year estimate, LB2019 is the lower bound of the uncertainty interval for the 2019 mid-year estimate, UB2019 is the corresponding upper bound, and xXB are the corresponding relative uncertainty bounds.
The relative uncertainty bounds for the merged LAs are calculated as a weighted average of those of its constituent LAs:
3.
Where xi is the relative uncertainty bound for LAi and wi is the proportion of the population in LAi and xT is the relative uncertainty bound for the merged LA. Equation 3 is calculated separately for the lower and upper bound of the mid-year estimate.
The relative uncertainty bounds for the 2020 mid-year estimates are then calculated as:
4.
5.
This was calculated for all local authorities in Table 1.
Changes to the international migration component
We use bootstrapping to simulate uncertainty around the international migration component, however this was not possible because of computational issues encountered in implementing the method. Instead, we use the international migration point estimate and uncertainty interval from 2011 to 2019 for each LA. This is to identify patterns in the widths of the intervals and the position of the point estimate in the interval over time. No specific patterns were found. Therefore, we calculate the relative width of the 2019 uncertainty intervals and apply to the 2020 MYE for the international migration component.
The estimates for the 2020 international migration component are based on the International Passenger Survey (IPS) data up to March 2020, and modelled migration estimates for the period after March 2020 when the IPS was suspended because of coronavirus (COVID-19). Figure 1 summarises the changes that have been made to produce the 2020 mid-year estimate uncertainty.
Figure 1: 2020 mid-year estimate cohort component method and statistical uncertainty
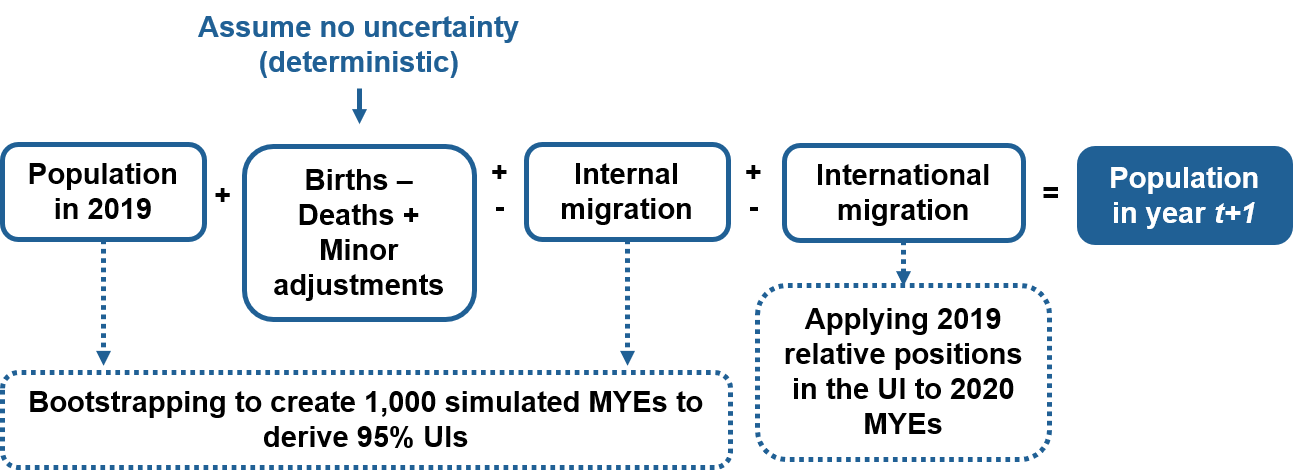
Source: Office for National Statistics
Download this image Figure 1: 2020 mid-year estimate cohort component method and statistical uncertainty
.png (30.7 kB)4. Location of the MYEs in their uncertainty intervals
We produce uncertainty intervals for all local authorities in England and Wales.
Table 2 shows that for most local authorities, the mid-year population estimates (MYEs) no longer sit within its uncertainty interval in 2020.
Over time, a growing number of local authority MYEs fall outside of their empirical 95% uncertainty bounds. By 2020, this is the case for 161 local authorities. This is consistent with our understanding that estimation of the population becomes progressively more difficult as we move away from the census.
| Year | Number within | % | Number above | % | Number below | % |
|---|---|---|---|---|---|---|
| 2011 | 348 | 100.00 | ||||
| 2012 | 347 | 99.71 | 1 | 0.29 | ||
| 2013 | 316 | 90.80 | 28 | 8.05 | 4 | 1.15 |
| 2014 | 271 | 77.87 | 66 | 18.97 | 11 | 3.16 |
| 2015 | 237 | 68.10 | 95 | 27.30 | 16 | 4.60 |
| 2016 | 218 | 62.64 | 108 | 31.03 | 22 | 6.32 |
| 2017 | 195 | 56.03 | 120 | 34.48 | 33 | 9.48 |
| 2018 | 187 | 53.74 | 123 | 35.34 | 38 | 10.92 |
| 2019 | 177 | 50.86 | 130 | 37.36 | 41 | 11.78 |
| 2020 | 161 | 47.92 | 125 | 37.20 | 50 | 14.88 |
Download this table Table 2: Position of local authority mid-year population estimates relative to their empirical 95% uncertainty intervals, 2011 to 2020
.xls .csvTable 3 outlines how the local authorities are interacting with the uncertainty interval. Figures 2 to 7 provide illustrative examples of local authorities for each position in this table.
| Position over time | Empirical 95% |
|---|---|
| MYE sits within the uncertainty interval | 76 |
| MYE drifts to upper bound | 44 |
| MYE drifts to lower bound | 34 |
| MYE crosses upper bound | 125 |
| MYE crosses lower bound | 47 |
| MYE follows none of these trends | 10 |
| Total | 336 |
Download this table Table 3: Position of local authority mid-year population estimates relative to their uncertainty intervals
.xls .csv
Figure 2: The mid-year population estimate sits within its uncertainty intervals, 2011 to 2020 – Boston
Source: Office for National Statistics – measures of statistical uncertainty
Download this chart Figure 2: The mid-year population estimate sits within its uncertainty intervals, 2011 to 2020 – Boston
Image .csv .xls
Figure 3: The mid-year population estimate drifts to the upper bound of the uncertainty intervals, 2011 to 2020 – Castle Point
Source: Office for National Statistics – measures of statistical uncertainty
Download this chart Figure 3: The mid-year population estimate drifts to the upper bound of the uncertainty intervals, 2011 to 2020 – Castle Point
Image .csv .xls
Figure 4: The mid-year population estimate drifts to the lower bound of the uncertainty intervals, 2011 to 2020 – Cardiff
Source: Office for National Statistics – measures of statistical uncertainty
Download this chart Figure 4: The mid-year population estimate drifts to the lower bound of the uncertainty intervals, 2011 to 2020 – Cardiff
Image .csv .xls
Figure 5: The mid-year population estimate crosses the upper bound of the uncertainty intervals, 2011 to 2020 - Mid Devon
Source: Office for National Statistics – measures of statistical uncertainty
Download this chart Figure 5: The mid-year population estimate crosses the upper bound of the uncertainty intervals, 2011 to 2020 - Mid Devon
Image .csv .xls
Figure 6: The mid-year population estimate crosses the lower bound of the uncertainty intervals, 2011 to 2020 – Cheltenham
Source: Office for National Statistics – measures of statistical uncertainty
Download this chart Figure 6: The mid-year population estimate crosses the lower bound of the uncertainty intervals, 2011 to 2020 – Cheltenham
Image .csv .xls
