1. Introduction
In simple terms, productivity is a measure of how much output is generated per unit of input. It is important because higher productivity is well understood to be linked to higher incomes and standards of living. However, differences in the way that output and inputs are defined lead to various approaches to measuring productivity, which can make comparisons between the approaches difficult.
The Office for National Statistics (ONS) is a world leader in measuring productivity, producing three distinct sets of routine estimates:
- Labour productivity (L-prod)
- Multi-factor productivity (MFP)
- Public service productivity (PSP)
Also included in the MFP datasets are capital productivity (K-prod) series.
This article explains the important differences between these statistics, and how to correctly interpret them. They show systematically different growth rates, particularly public service productivity (PSP), which can in part be explained by the methodologies used to derive them. This article explains their similarities and differences so users can see how the series can and cannot be used together.
Labour productivity
Labour productivity (L-prod) is the simplest of the productivity measures that the ONS publishes, in conceptual terms. It divides output, as measured on a gross value added (GVA) basis, by the quantity of one input factor (labour) to give the amount of output per hour worked, or sometimes per worker or per job.
It is the most recognised and well-known of the measures, and data from the series were used to calculate the average annual increase in UK productivity in the past decade, which was the Royal Statistical Society’s UK Statistic of the Decade. It is widely referenced and commented on by users across government, industry and the media. See the quality and methodology report for more details.
Multi-factor productivity
Multi-factor productivity (MFP) is closely related to L-prod, but takes account of two input factors, labour and capital, to identify how much growth in each contributes to the growth in GVA output. Importantly, any residual is allocated to a third variable, MFP itself – also often called “technology” or the “Solow residual” after Robert Solow: the economist who identified it. This element accounts for changes in output that cannot be explained by a change in measured inputs.
The measure of labour inputs used is also slightly different from that used for L-prod. L-prod assumes all hours of labour input to be equivalent. The MFP analysis applies an adjustment to reflect the quality of the labour input, broadly proxied using wage rates. See the quality and methodology information report for more details or the simple guide to MFP.
K-prod is a twin to L-prod, but it uses the measure of capital from MFP rather than labour inputs as the denominator to derive a unit of output per unit of capital, in the same way that L-prod gives the unit of output per unit of labour (hour worked).
L-prod and MFP both illustrate the UK’s “productivity puzzle,” the maintained weak growth of productivity in the UK since the economic downturn of 2008 – with the Office for National Statistics (ONS) regularly contributing to the debate.
In the quarterly MFP bulletin, the L-prod series is decomposed into the drivers in our MFP calculations; that is, labour composition, capital deepening (the amount of capital per worker) and the MFP residual, showing technological gains among other factors. This helps our users better understand the relationship between the two measures.
It is also useful to note that L-prod is produced for the whole economy and the market sector, and MFP is produced for the market sector only, with industry breakdowns available for both.
Public service productivity
The third measure, public service productivity (PSP), is more distinct, both methodologically and in terms of scope, which makes it difficult to compare with other measures of productivity.
It covers publicly-funded services (irrespective of whether these are delivered by public or private sector providers). Importantly, the state provides them “free at the point of use”, so there is no market price for the output. Therefore it is difficult to derive an equivalent measure of GVA. GVA removes intermediate inputs from gross output to give the marginal contribution of the industry to the economy, but without prices for the output in PSP it is hard to remove the input component.
As such, PSP can be described as being a “total output MFP” measure; accounting for labour, capital, and intermediate inputs as inputs, rather than a “GVA MFP” measure, which removes intermediate inputs from output to generate GVA. This is illustrated in Figure 1 – note the inclusion of the Solow residual (which accounts for changes in output not explained by a change in inputs) for MFP and for PSP.
Figure 1: How the different productivity measures are constructed
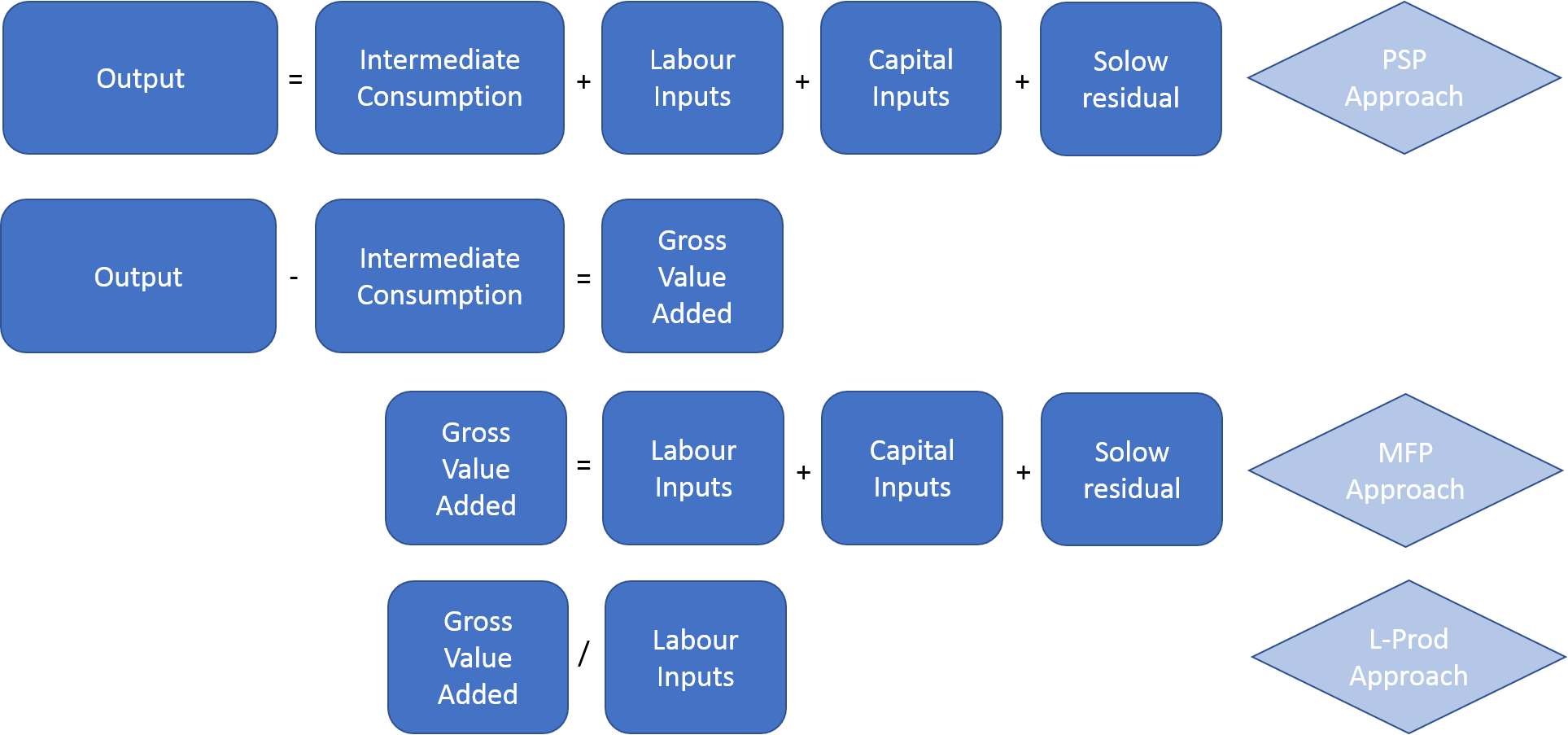
Source: Office for National Statistics
Notes:
- This diagram serves to only summarise and visualise the components of the productivity measures. The additivity of the components is only possible within the growth accounting framework for MFP and PSP, which are both calculated as changes.
Download this image Figure 1: How the different productivity measures are constructed
.png (51.2 kB)Table 1 illustrates the fundamental differences between these statistics. Definitions of main terms are provided in Section 7.
| Labour productivity | Multi-factor productivity | Public service productivity | |
|---|---|---|---|
| Inputs | Labour – hours worked, number of jobs, or number of workers | Labour – quality-adjusted labour input (QALI) | Labour – full-time equivalents, or implied volume of labour |
| Capital – volume index of capital services (VICS) | Capital – consumption of fixed capital | ||
| Intermediates – deflated expenditure on intermediate goods and services | |||
| Output | Gross value added | Gross value added | Output, as measured by a cost-weighted activity index with quality adjustment |
| Coverage | Whole economy, market sector, and industry breakdowns: using SIC | Market sector, and industry breakdowns: using SIC | Total public services, and service area breakdowns: using COFOG |
Download this table Table 1: Important differences in inputs and output for ONS’s three productivity measures
.xls .csvGiven their differing measurement approaches, different types of productivity have different interpretations. The rest of this article provides an overview of the uses of productivity but is far from exhaustive. Interested readers are encouraged to refer to the OECD Measuring Productivity Manual, particularly Chapters 2 and 10 (PDF, 994KB), the ONS Productivity Handbook (PDF, 3.2MB), and Oulton (2020) (PDF, 1.0MB).
Nôl i'r tabl cynnwys2. Inputs
For labour productivity (L-prod), as shown in Table 1, labour is the only input factor – either total hours worked, number of jobs, or number of workers. The headline measure is output per hour, so the main input factor is hours worked.
For multi-factor productivity (MFP), quality-adjusted labour inputs (QALI) are used, which account for changes in the composition of the workforce, giving higher weight to more skilled workers. This is in addition to measuring changes in hours worked.
The capital input term is a volume index of capital services (VICS), reflecting the flow of services received rather than the value of the capital stock. For example, a construction firm may use certain software for design purposes; capital services measure the design service that the software produces, as that service is an input to produce the final output. Quality and methodology reports are available for both QALI and VICS.
As stated in Section 1, the MFP calculation decomposes growth in GVA (gross value added, an output measure) into changes in labour, capital and a residual MFP term.
For public service productivity (PSP), labour, capital, and intermediate inputs are used. Nine service areas1 are included in the estimates; for most, inputs are measured indirectly by using deflated current expenditure. Where possible, inputs are measured directly, such as using the number of full-time equivalent staff.
This means that whilst each method is trying to deliver a similar result, there are subtle differences between them, which can make comparisons complex. To disentangle this aspect, we suggest two examples, focusing on labour quality and technical change.
Example 1: labour quality
MFP accounts for changes in labour quality through its use of QALI as its labour input. PSP accounts for changes in labour quality through, where possible, counting different types of workers (for example, teachers and teaching assistants), and weighting them together using salary data.
L-prod does not account for labour quality in any of its labour inputs – therefore, if the workforce was to become more skilled, the resulting positive effect on output (assuming all else is equal) would show as an increase in the estimate of labour productivity. In this case, L-prod growth would be greater than MFP and PSP growth, as the latter both isolate the effect of the improved skills of the workforce in their labour inputs.
Example 2: technical change
Technical developments can be linked to a specific input (embodied) or they can be independent of the inputs (disembodied). Technical change could be linked to capital or intermediate inputs.
For capital, a machine in a factory could have its working day extended by the fitting of a new, tougher part. For intermediate inputs, semiconductors are an important intermediate input to computers, therefore any improvements in the quality of semiconductors would result in a change in quality of the computers. These are examples of embodied changes.
Technical change may also be more widely cast, such as an improvement to organisational efficiency – this might affect all aspects of the production process, not just that aspect that utilises capital inputs, for example. This is an example of disembodied change.
Disembodied change is captured in all three estimates of productivity because we cannot isolate it via an input factor. Embodied change for capital is isolated from final estimates of PSP and MFP as capital is measured as its own input (but only as far as the quality of the deflators used allows). The same is true in the case of PSP and intermediate inputs.
These concepts are summarised in Table 2.
| What is included in the productivity estimate? | Labour productivity (based on GVA) | Multi-factor productivity (based on GVA) | Public service productivity |
|---|---|---|---|
| ‘True’ productivity/Solow residual | ✓ | ✓ | ✓ |
| Labour quality: changes in personal capacities/skills of workers | ✓ | ||
| Embodied technical change: changes in fixed capital | ✓ | ||
| Embodied technical change: changes in intermediate inputs | ✓ | ✓ | |
| Disembodied technical change: organisational efficiency, economies of scale, capacity utilisation, spill-overs, etc. | ✓ | ✓ | ✓ |
| Measurement errors | ✓ | ✓ | ✓ |
Download this table Table 2: What the different productivity estimates may capture
.xls .csvThese differences are explained in greater depth in the OECD Measuring Productivity Manual (PDF, 994KB), specifically Chapter 2, which explains the interpretation, and advantages and limitations of each type of productivity measure. This all has one last implication. The fewer input factors, the greater the potential variation in the final productivity estimate and the higher the implied rate of productivity growth. To simplify this, we might deduce that in growth rate terms (for the same organisation, industry, sector, or economy):
L-prod > MFP > PSP
To summarise, isolating growth in an input factor helps to explain the cause of productivity growth – you could also call them “control variables”. Because L-prod has fewer explanatory “control variables”, any changes in productivity that these would have captured get rolled into the headline estimate. This naturally makes it larger (assuming all productivity impacts are positive) than MFP and PSP, which use more “control variables”.
MFP and L-prod do both use a GVA measure for output, which is output minus intermediate inputs. However, this does not have the same effect as explicitly accounting for intermediate inputs as PSP does. For more detail on this concept, called the “productivity ratio” in this article, see Section 5. (Also explained in Section 5 is the importance of GVA and total output measures in their own right in determining levels of growth.)
The differences in the inputs used by each type of productivity measure make comparisons of productivity growth between the three difficult. However, they also vary in terms of output measures and coverage.
Notes for Inputs:
- These services areas are: healthcare; education; public order and safety; adult social care; children’s social care; social security administration; defence; police; and other government services.
3. Output
In the national accounts, gross value added (GVA) is generated by removing intermediate inputs from measures of output. GVA is used in labour productivity (L-prod) and multi-factor productivity (MFP). However, as MFP only covers the market sector, a “market sector proportion” of GVA is used – for industries that have some non-market element, GVA between MFP and L-prod is different. In public service productivity (PSP), output is not measured by GVA, but instead uses the national accounts concept of output.
The actual method to derive the output measure for PSP is described as a cost-weighted activity index; where possible, the relevant activities undertaken as part of delivering the service are measured and aggregated together using cost weights. For more information see Sources and methods for public service productivity estimates. This method is necessary because of the lack of market prices in the provision of public services.
For some service areas, it is difficult to measure output directly. Three areas (police, defence and other government services) are measured fully using the “output-equals-inputs” convention – here it is assumed output growth is equal to inputs growth. We also use this convention for a proportion of output for children’s social care, adult social care and healthcare. As a result, a proportion of PSP sees no measured productivity change. For 2017, the latest year in which data are available at the time of writing, “output-equals-inputs” applies to 41% of total output.
PSP also uses quality adjustment techniques, unique to these statistics. These are used to assess changes in the quality of the services provided. In the market sector of the economy, assuming competitive markets, changes in prices signal changes in quality, and as stated there are no market prices in public services. These quality adjustments are consistent with System of National Accounts 2008, but European System of Accounts 2010 (ESA 2010) does not permit these.
Office for National Statistics estimates of GVA1, which are used in L-prod and MFP, do not include this quality adjustment, as these are produced on an ESA 2010-consistent basis. These data, contained in the national accounts, are therefore less suitable measures of productivity for the non-market sector. For more details see the guide to quality adjustment.
The ways in which data are collected for market and non-market output can also contribute to making comparisons difficult – for example, consider healthcare, which has market (private) and non-market (NHS) activity in the UK. Healthcare is industry Q (human health and social work activities) in the Standard Industrial Classification system. Healthcare is also the largest of the nine service areas measured in PSP and is published in its own annual articles on a calendar year, UK basis and a financial year, England basis as well as in the total public service productivity estimate.
For healthcare market output, a deflated turnover series is used, where current price turnover data is collected from the Monthly Business Survey. Monthly turnover estimates give an early indication of what is happening in the economy, as turnover is an appropriate output proxy indicator. This current price turnover data is deflated using the Consumer Prices Index for healthcare to derive a volume estimate.
For non-market output, data from a variety of administrative sources are used to estimate the volume of activities such as hospital and ambulance services, community care and general practice activities. Details on healthcare measurement for PSP are included in Sources and methods for public service productivity estimates: healthcare (PDF, 328KB). Data are largely from the Department of Health and Social Care and its devolved counterparts.
These two methods of collecting data could mean that reconciling the two is difficult. Administrative data is that collected in day-to-day work, while surveys are designed for a more specific purpose, and there could be subsequent differences in the type of data included.
The differences in measuring output using the output and GVA approaches complicate comparisons between PSP and other productivity measures, especially when quality adjustments are also factored in. A final point of interest is that PSP includes inputs of goods and services that are outputs of the market sector, and while the market sector does not directly purchase outputs as identified in PSP, it does implicitly use healthcare, education, publicly provided infrastructure, and so on. This overlap between inputs and output between the market and non-market sectors complicates the relationship between PSP, and MFP and L-prod.
Notes for: Output
- Despite the conceptual differences between GVA and output, one should also note that for practical reasons, output is used as a proxy for GVA in the short-term in the national accounts. GVA is used only once the balancing process of supply and use tables is complete, with a time lag of around three years – more detail is in Section 5.
4. Coverage
As well as methodology, Table 1 showed that there are differences in coverage between the three sets of productivity estimates; they measure different activities and parts of the economy.
Labour productivity (L-prod) and multi-factor productivity (MFP) both show the productivity of the market sector. They also both provide industry breakdowns using the Standard Industrial Classification (SIC) system. L-prod is also produced for the whole economy, arguably the most important measure for users, and public service productivity (PSP) measures public services only.
The closest approximation of public services included in SIC are industries O, P and Q, which are public administration and defence, education, and human health and social work activities respectively. These are often collectively referred to as “government services” industries. They include a large proportion of the same activities as PSP, but not all. They also include market activities in these industries, such as private education, and some public services are omitted from them, such as waste services. Therefore, they cannot be compared with PSP measures, although they do offer the closest approximation to PSP available within the L-prod or MFP frameworks.
Table 3 visualises this relationship. Government final consumption expenditure is published by the Office for National Statistics and is the basis for measuring output in PSP. More information on output is in Section 3. PSP uses Classifications of the Functions of Government to classify activities rather than SIC.
| GDP Expenditure framework | |||
|---|---|---|---|
| Government final consumption expenditure (total public services) | Other expenditure | ||
| GDP Production framework | OPQ produced (Government services) | E.g. state schools, police services, healthcare | E.g. private education, private healthcare |
| Non-OPQ produced | E.g. waste management, water supply, libraries and museums | Other consumption, investment, net trade, etc. | |
Download this table Table 3: Conceptual link between public services provision and government services production
.xls .csvTable 3 is taken from Comparing public service productivity estimates with other productivity estimates (PDF 434KB) by Gill and Kliesmentyte, formerly of ONS. The information note was published in June 2015 and explains the differences in output coverage in more detail.
Nôl i'r tabl cynnwys5. The productivity ratio
The previous sections of this article explained how differences in the coverage of the three productivity approaches produced by the Office for National Statistics (ONS), and the methodology used for inputs and output for each, complicates comparisons. However, productivity growth will also differ between public service productivity (PSP) and the GVA (gross value added)-based productivity measures because of differences in the relative coverage of the inputs and output sides of the productivity ratio.
Value added, as a statistical concept, is a share of the output produced – it shows the productive capacity of the business, industry, economy and so on. It is affected by the prices of inputs and output, as well as technical progress. It can show technical progress for the inputs it accounts for – so labour and capital for multi-factor productivity (MFP) – but not any progress if it affects other input factors (that is, intermediate inputs for MFP).
Value added is in fact dependent on the prices of these intermediate inputs – if prices increase, producers either pay more or use less, which affects the final value of GVA. For output measures, intermediate inputs appear on the inputs side of the equation and do not affect output in this way. Technical changes for labour, capital and intermediate inputs are all isolated and only disembodied technical change remains – this is the case for PSP.
Notation can be used to express this.
Taking Y = f(K,L,IC), where Y = output, K = capital, L = labour and IC = intermediate consumption (another term for intermediate inputs),
in MFP:
whereas in PSP:
Intermediate consumption is only on the right-hand side for PSP, as an input factor. As MFP and PSP measure different parts of the economy, no equivalence is possible between the two production functions. It could be suggested that, if the same parts of the economy were measured, and if δ = 1, then intermediate consumption could be carried to the left-hand side of the PSP equation, and as Y – IC = GVA, in this case γ MFP would equal γPSP1. This is not true for two practical reasons.
Firstly, in the national accounts, GVA at the industry level is derived from a gross domestic product (GDP) level set by the expenditure method. As discussed in Martin Weale’s forthcoming contribution to our essay series, whilst this sets the economy-wide level of GDP using a “double-deflation” approach, currently industry level results may not be fully consistent with a double-deflated output measure, instead reflecting output patterns. This is true everywhere except the public services, which by including inputs in the right-hand side of the equation deliver double-deflated output measures.
Therefore, while MFP results at the industry level may change as a result of double-deflation, PSP results are likely to remain the same. There are also certain practicalities about GVA measurement to consider2.
Secondly, following a little manipulation of the previous formula, it can be observed that the rate of change of MFP with GVA on the left-hand side equals the rate of change of MFP with output on the left-hand side, multiplied by the inverse of the nominal share of value added in output. Given intermediate consumption is always positive, GVA as a share of output must be less than one.
As the inverse of a number less than one has to be greater than one, it is clear that an equation constructed with GVA on the left-hand side will systematically grow at a faster pace than one with output on the left-hand side. As such, the method applied in MFP, if it were applied to the same data, would deliver a faster growth rate in the Solow residual than the method applied in PSP.
Assuming certain economic conditions, such as competitive markets, and crucially also assuming that all sectors have a similar underlying growth rate of productivity, then typically we could expect growth by the way of L-prod> MFP> PSP. This was also stated in Section 2, using the idea of input factors acting as “control variables”. This result is complex and the previous description presents an extremely simplified rendition of the problem. As will be demonstrated later, in some time periods this bears out but not in others.
More information and a mathematical proof can be found in Bruno (1978) (PDF, 462KB). This is summarised and further explained in the OECD Measuring Productivity Manual (PDF, 994KB) and Phelps (2010) (PDF, 253KB).
As stated previously and throughout this article, ONS productivity measures produced using the MFP and PSP approaches cannot be easily compared. There are important differences between the two measures in the methodologies employed. However, all productivity measures spring from the same basic set of methods, and while the obvious caveats need to be respected, as long as they are understood, approximate comparisons could be made. These comparisons function primarily as a thought exercise and not a basis for policy.
Notes for productivity ratio
This is also very difficult in practice as the parameters between the two functions are very different, as would be the weighting (alpha and beta are different between PSP and MFP as denoted by the subscripts). Also note that changes in the variables have been used, as per growth accounting standards.
The methodology used to account for intermediate consumption in GVA is not necessarily comparable with how estimates of intermediate inputs are produced in the PSP framework, and differs depending on the timeframe studied. Over the longer term, supply-use balanced data are used to calculate GVA. In the supply use balanced period (at the time of writing, for 1997 to 2016), current price data are benchmarked at industry level to GVA produced as part of the supply use balancing. The supply use framework is used to achieve consistency by linking the components of GVA, inputs, outputs and final uses. In the short run (at the time of writing, 2017-onwards), output is measured as a proxy for GVA, because of the practicalities of data collection. For intermediate inputs in PSP, administrative data are used rather than business surveys, and there are no long- or short-term changes in the methodology. There are, however, regular revisions to these data.
6. How the three productivity measures compare
This article has so far explained numerous reasons why a direct comparison of public service productivity (PSP) with labour productivity (L-prod) or multi-factor productivity (MFP) is difficult because of differences in methodology and coverage. These various differences must be clearly understood before attempting a comparison.
Observing the growth rates of the three types of productivity does illustrate some of the concepts described previously. The growth rates are shown in Figure 2. For PSP, the series used only includes service areas with direct output measurement so that change in productivity is measured – the service areas defence, police and other government services are excluded.
Quality adjustment is a unique technique in PSP; however, the quality adjusted series has been used for these charts because in L-prod and MFP, the use of market prices means that changes in quality are accounted for. Note that data for PSP for 2018 and 2019 are adapted from our quarterly, experimental estimates and therefore do not include any quality adjustments.
Figure 2: Public service productivity shows a different trend to the rest of the economy
Labour productivity, market sector labour productivity, multi-factor productivity and public service productivity (for directly measured service areas only), UK, 1997 to 2018
Source: Office for National Statistics
Notes:
- The public service productivity estimates for 2018 and 2019 are based on experimental quarterly statistics unlike the rest of the series, including no quality adjustments. The definitions of the service areas used are also different in the quarterly estimates; they are health, social protection, education and justice and fire, whereas for the annual data the areas are healthcare, education, adult social care, children’s social care, social security administration, and public order and safety.
- The data used in this chart are from the following sources: L-prod from Labour productivity: Tables 1 to 8 and R1; MFP from Multi-factor productivity estimates; PSP from Public service productivity estimates: total public service and internal calculations based on Public service productivity: quarterly, UK, October to December 2019
Download this chart Figure 2: Public service productivity shows a different trend to the rest of the economy
Image .csv .xlsFirst, the profile of the L-prod and MFP measures are similar, both growing steadily up until 2007, falling in the subsequent years, and rebounding slowly since 2009 with some years of decline. This trend is the “productivity puzzle”, as introduced at the start of this article.
MFP only covers the market sector, but as this makes up the majority (around three-quarters) of the whole economy, it is no surprise they show similar trends. Indeed, the L-prod measures for the whole economy and market sector are very similar. As noted previously, the growth rate of MFP is lower than for L-prod, even for the same1 part of the economy (the market sector). This is because growth in L-prod will capture improvements in input factors that MFP accounts for, such as changes in the quality of labour and the increased use of capital inputs.
By contrast, public service productivity (PSP) changes more slowly up until 2010 (with years of growth and decline) and consistently rises more rapidly since then. It may be expected that public service productivity would grow more slowly than a L-prod or MFP measure for public services, from a conceptual standpoint, as described in Sections 2 and 5.
Figure 3 shows the same data as in Figure 2, but re-indexed to 2009 to better examine the effect of the economic downturn on the productivity series.
Figure 3: Since the financial crisis, MFP growth declined more than PSP and Lprod, with the market sector as a whole falling more
Labour productivity, market sector labour productivity, multi-factor productivity and public service productivity (for directly measured service areas only), UK, 2009 to 2018
Source: Office for National Statistics
Notes:
- The public service productivity estimates for 2018 and 2019 are based on experimental quarterly statistics unlike the rest of the series, including no quality adjustments. The definitions of the service areas used are also different in the quarterly estimates; they are health, social protection, education and justice and fire, whereas for the annual data the areas are healthcare, education, adult social care, children’s social care, social security administration, and public order and safety.
- The data used in this chart are from the following sources: L-prod from Labour productivity: Tables 1 to 8 and R1; MFP from Multi-factor productivity estimates; PSP from Public service productivity estimates: total public service and internal calculations based on Public service productivity: quarterly, UK, October to December 2019
Download this chart Figure 3: Since the financial crisis, MFP growth declined more than PSP and Lprod, with the market sector as a whole falling more
Image .csv .xlsFigure 3 shows that the market sector appeared to fare worse from the economic downturn in 2008. L-prod for the market sector declines the most in 2012 and 2013 (and whole economy L-prod also declines in 2012), and MFP experiences this same fall in growth but recovers more quickly. However, by the end of the series, MFP and L-prod converge to reach similar cumulative growth rates. PSP, in contrast, outgrows the other measures since 2013, having not experienced the same decline around that time.
Following a small decline in 2010, PSP has an average annual growth rate of 0.9% between 2010 and 2017. This compares with an average annual growth of negative 0.1% between 1997 and 2009. In 2018, we see another fall in PSP – however, this is based on experimental quarterly estimates, which have methodology differences with the 1997 to 2017 National Statistic series including no quality adjustments. More details on the trend in PSP growth since the downturn is in Public service productivity: total, UK, 2017 (the latest data at the time of writing).
PSP’s higher growth in the past years counters the assumption outlined previously where PSP may be expected to grow more slowly than other measures, as it accounts for more “control variables”. However, these variables are only one part of the picture – this article has also discussed important differences in output measurement and coverage. As the L-prod series for the market sector fell the most, it could be concluded that differences in coverage are vital here.
The trend rates of growth in Figure 2, with PSP appearing to have a much lower growth rate than the others, are because of the much faster growth in the other measures before the downturn – perhaps another facet of the productivity puzzle.
However, as this article has explained, making this comparison between PSP and the other measures is difficult. While an interesting exercise, further use in analysis or policy should be done with caution. There are numerous reasons why this is the case.
These reasons include:
- MFP and PSP both account for capital inputs, and all three account for labour inputs, but PSP measures these in a different way to the others
- PSP alone explicitly accounts for intermediate inputs
- PSP uses a total output measure rather than gross value added (GVA); it has been shown that GVA-based measures will grow faster than total output-based measures (under certain conditions)
- PSP output, in turn, uses the “output-equals-inputs” convention for around 41% of all public services (meaning that there is no measured productivity at all) and uses quality adjustment for around 51% (with some small overlap between these two) – neither of these are used for the other productivity measures
- PSP has a very different coverage
Notes for: How the three productivity measures compare
- Calculation of the market sector differs slightly between our labour productivity and MFP estimates. We intend to align these in future releases.
7. Important definitions
A number of important terms and concepts are used throughout this article. Often, they have similar meanings, with important differences. The differences in sectors are nuanced and are explained briefly here. For more detail, please see the European System of Accounts (ESA) 2010 (PDF, 6.4MB), in particular Diagram 2.1. For detail on where the Office for National Statistics classifies certain bodies in the UK, see the public sector classification guide.
Total output
The total value of products created in the period. Equivalently, it is the total value of sales of products (or turnover) in the period, and changes in inventories held. It includes market output, output produced for own final use, and non-market output, and incorporates the costs of intermediates used to make the output. It is gross of depreciation – that is, it is value before the depreciation of the capital goods used in production has been deducted.
Gross value added
GVA is total output minus intermediate consumption (the cost of intermediate inputs used up in the production process, such as raw materials, energy, utilities, and business services). It is also gross of depreciation.
Private sector
One of the institutional sectors of the economy, made up of all bodies and companies that are not controlled by government. This includes private corporations (financial and non-financial), and non-profit institutions serving households (NPISH).
Public sector
One of the institutional sectors of the economy, made up of all bodies and companies that are controlled by government. This includes government (both central and local) and public corporations – companies that operate in the market (for profit) but are at majority controlled by government. The complement of the private sector.
Market sector
The part of the economy thatcharges economically meaningful prices for its output, and as such operates for profit. Includes all profit-making corporations, whether privately or publicly funded, and excludes non-profit institutions serving households (such as charities) and general government.
Non-market sector
The part of the economy that does not sell its output for economically meaningful prices, and as such does not operate for profit. Includes government and non-profit institutions serving households (NPISH) operating in the private sector.
Public services
Services delivered for the public good from public expense. Includes all methods of public service delivery, not only services provided directly by public bodies. For instance, healthcare services provided by a private hospital, funded by the NHS, would be included in this measure.
Government
Consists of organisations that are non-market producers whose output is intended for individual and collective consumption and are financed by compulsory payments (such as taxes) made by bodies belonging to other sectors. Also includes bodies principally engaged in the redistribution of national income and wealth.
Government services industries
Industry sections O (public administration and defence), P (education) and Q (health and social care) of the Standard Industrial Classification (SIC) 2007. These are often collectively referred to as “government services” industries, or OPQ.
| Sector | Private or public? | Market or non-market? |
|---|---|---|
| Public non-financial corporations | Public | Market |
| Private non-financial corporations | Private | Market |
| Private financial corporations | Private | Market |
| Public financial corporations | Public | Market |
| Government (central and local) | Public | Non-market |
| Non-profit institutions serving households (NPISH) | Private | Non-market |
